Funding
-
Finite topomorphic extensions of equicontinuous systems (DFG research grant)
DFG Logo
Graphic: DFGUnderstanding and quantifying the dynamical complexity of time-dependent systems is one of the major goals of Dynamical Systems Theory. Thereby, a central role is played by the notion of entropy, which allows to measure the ‘chaoticity’ inherent in a system. However, even when entropy is zero, one may find a remarkable variety of dynamical behaviours and many weaker forms of chaoticity. Hence, the study of zero-entropy systems is a subject of independent interest and has seen considerable activity over the past decades. A particular motivation for these endeavours comes from the theory of aperiodic order, where mathematical models of quasicrystals and other aperiodic structures with long-range order, such as the Penrose tiling, induce associated dynamical systems. Different degrees of long-range order then correspond to different levels of dynamical complexity.
An alternative viewpoint is to study dynamical systems via extension structures. This approach has led to seminal results such as the Furstenberg Structure Theorem for distal flows, the Furstenberg-Zimmer Structure Theorem for measure preserving flows or the Veech Structure Theorem for point-distal flows. In the context of low-complexity systems, one may hope to characterise important system classes by basic extension structures. For instance, there has recently been considerable progress in the understanding of mean equicontinuous minimal systems. Glasner and Downarowicz showed that these are isomorphic to their maximal equicontinuous factor (MEF), an underlying compact group rotation which encodes the `regular' (non-chaotic) part of the dynamics. Subsequent results established a hierarchy of subclasses, characterised by different invertibility properties of the factor map to the MEF.
The aim of the project is to build on these recent advances and extend the theory to a more general class of systems – referred to as finite-to-one topomorphic extensions. This provides a natural next step in the understanding of low- complexity dynamics from the viewpoint of extension structures. Moreover, this class comprises a broad scope of systems of both theoretical and practical interest, including paradigmatic examples in the field of aperiodic order, like the Thue-Morse or Rudin-Shapiro subshifts. The first objective of the project is to provide dynamical characterisations of finite-to-one topomorphic extensions and important subclasses, defined again in terms of structural properties of the MEF factor map. Further dynamical properties will then be related to the classification. In order to measure the complexity of finite-to-one topomorphic extensions, we intend to introduce a sequence of taylor-made topological invariants, generalising the recently introduced notion of amorphic complexity. Moreover, the construction and investigation of novel examples will play a prominent role and go hand in hand with the development of the general theory.
-
Dynamical spectra of irregular Toeplitz flows (CSC Phd fellowship Zeyu Kang)
MSc Zeyu Kang has been awarded a 4-year Phd fellowship by the Chinese Scholarship Council to work on the spectral theory of dynamical systems and its applications to irregular Toeplitz flows and other example classes from symbolic dynamics and aperiodic order (mathematical quasicrystals).
Project CSC N° 202308080192, started 01/24.
-
Topological Dynamics and Spectral Theory of Extensions of group rotations (PhD Fellowship Lino Haupt, German Academic Scholarship Foundation)
MSc Lino Haupt has been awarded a 2-year PhD fellowship by the German Academic Scholarship Foundation to work on topological dynamics and spectral theory of extensions of group rotations.
Starting 07/25.
-
Entropy theory in the context of aperiodic order (Studienstiftung, PhD fellowship, Till Hauser), 2019-2020
MSc Till Hauser has been awarded a PhD fellowship by the German Academic Scholarship Foundation (Studienstiftung des deutschen Volkes), allowing him to study entropy theory in the context of general group actions and to apply the results to the dynamics of mathematical models of quasicrystals.
-
Anosov diffeomorphisms and hyperbolic dynamics (Capes-Humboldt postdoc fellowship, Bernardo Carvalho), 2018-2020
Dr. Bernardo Carvalho has been awarded a 2-year postdoctoral fellowship by CAPES and the Alexander von Humboldt foundation to work on the dynamics of Anosov diffeomorphisms, with a patricular focus on topological hyperbolicity, shadowing properties and the existence of global product structures.
-
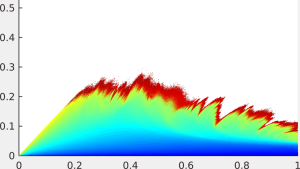
Surface dynamics (DFG individual project), 2017-2021
Parameter space of the kicked Harper map
Image: Tobias JägerIn dynamical systems theory, the understanding of low-dimensional systems is of major importance, since this often allows to elucidate and describe basic mechanisms and paradigmatic examples that prove to be relevant in a much broader context. While the understanding of one-dimensional systems is fairly complete and one of the great success stories of the field, two-dimensional systems are far less well-understood and fundamental problems in this area are still wide open.
The aim of the project was to make use of recent developments and elaborate new tools that have become available in the last years in order to address a number of central problems in surface dynamics. The main focus lies on the classification of zero entropy systems, the transition to chaos and the rotation theory on two-dimensional manifolds. More specifically, results included a generalisation of a recent classification of area-preserving C-infinity diffeomorphisms of the sphere with zero entropy by Franks and Handel and progress on a conjecture by C. Tresser from 1983 on the occurrence of period doubling cascades on the boundary of chaos in surface dynamics.
Unforseen progress was made in the understanding of the onset of diffusion in the kicked Harper modelExternal link, a study that is motivated by different phenomenons in physics.
-
Heisenberg professorship (DFG): Topological, geometric and probabilistic aspects of dynamical systems, 2015-2021
DFG Heisenberg Logo
Image: DFGThe professorship `Ergodic Theory and Dynamical systems' was initially funded by the Heisenberg program of the German Research Council. In this context, research was carried out on topological, geometric and probabilistic aspects of dynamical systems.
DFG projects 271404167External link, 407739711. External link
-
Innovative Training Network CRITICS (EU Horizon 2020), 2015-2020
Our group has been benefitting partner in the EU-funded ITN `Critical Transitions in Complex Systems (CRITICS)External link (Marie-Sklodowska-Curie grant agreement No 643073), coordinated by Jeroen LambExternal link and Martin RasmussenExternal link (Imperial College London).
CRITICS Logo
Graphic: Chris Richley -
Stability and mode-locking for quasiperiodically forced systems (Humboldt postdoc fellowship, Jing Wang), 2014-2016
Dr. Jing Wang has been awarded an 18-month postdoctoral fellowship by the Alexander von Humboldt foundation to work on the dynamics of low-dimensional quasiperiodically forced systems, with a patricular focus on rotation theory, mode-locking and non-uniform hyperbolicity.
-
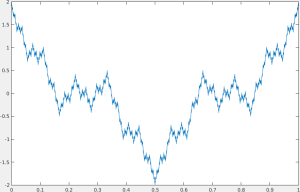
Scientific Network (DFG): Dynamics of skew product systems and multifractal analysis, 2012-2016
Graph of the Weierstrass Function.
Picture: Tobias JägerThe aim of the scientific network “Skew product dynamics and multifractal analysis” was to bring together experts on dynamical systems theory and fractal geometry in order to work on the interface of the two topics and to advance the state of the field, with a particular focus on the fractal strucutes of invariant curves and graphs-like repellers in skew product systems. A key element for the interaction within the network was a workshop program that has been implemented as a part of the project, with four major workshops organised on an annual basis in the years 2012–2015. Due to the partial school-like character of these events, they specifically fostered the development of several young scientists participating in the network and allowed them to acquire profound background knowlegde on various issues of high topicality. At the same time, the meetings allowed for a stimulating exchange on the ongoing research of the participants, and numerous publications were initiated at or benefited from these events.
During the project, substantial progress was made on several important aspects of the topic. For instance, a 25 year old conjecture on the fractal structure of so-called strange nonchaotic attractors has been confirmed, and methods have been established to determine the dimensions of such attractors in broad classes of systems. Further, members of the network have taken an active role in recent advances in the study of the graph of the classical Weierstrass function. The latter had been introduced by Weierstrass already around the beginning of the 20th century as an example of a continuous, but nowhere differentiable curve. However, a precise description of its fractal structure and dimensions has only been completed very recently. Further topics on which several advances were made include the study of bifurcation pattern and blowout bifurcations in forced systems and the fractal structure of so-called hyperbolic graphs, which occur in skew product systems with chaotic base dynamics and contracting fibres. Finally, surprising connections have been discovered between skew product dynamics and the mathematical theory of quasicrystals, and a substantial and fruitful transfer of ideas and methods between the two fields has been initiated. Further and more detailed information can be found in the final reportExternal link.
DFG project 217182598External link. Started 2012 at TU Dresden, transfered to Jena in 2015.
-
Emmy Noether project (DFG): Aspects of low-dimensional and non-autonomous dynamical systems, 2009-2016
Logo DFG Emmy Noether
Image: DFGErgodic Theory and Dynamical Systems have found their applications in basically all sciences and many other fields of mathematics. The aim of this research project is to make substantial contributions to this field, by analysing several types of low-dimensional and/or non-autonomous dynamical systems. A common feature of the systems under consideration is the lack, or irrelevance, of periodic orbits. This renders their investigation particularly difficult, since in the vast majority of situations where the long-term behaviour of a dynamical system is well-understood, the existence of periodic orbits is a crucial feature and often the starting point of any analysis. In contrast to this, periodic point free dynamics are still rather poorly understood in general, such that new concepts and ideas are required for their description.
The issues which should be addressed in particular include the role of so-called strange nonchaotic attractors in the classification of quasiperiodically forced circle maps, nonautonomous bifurcation theory with a particular focus on the two-step scenario for the non-autonomous Hopf bifurcation, and the classification of the dynamics of toral homeomorphisms. Applications to physics and biology, which shall be considered likewise, include the description of multi-frequency forced oscillators and neuronal coding under the influence of external noise.
DFG-Projekt 129048169External link. Started 2009 at TU Dresden, transfered to Jena in 2015.